Maths
Why do we teach Maths at Freeland Primary School?
We teach our children maths because it is an essential, lifelong learning skill. As well as numeracy, it helps skills such as problem solving, understanding and using shapes and measure and developing their own spatial awareness. Children can underperform in Mathematics because they think they can’t do it or are not naturally good at it. We want to ensure that we address these preconceptions by ensuring that all children experience challenge and success in Mathematics by developing a growth mind-set. At Freeland we want every child to feel like they are a maths learner and have the appropriate skills to tackle real life problems by the time they leave our school. Teaching children efficient and compact problem solving methods enables them to access further learning, increases their career options and improves their understanding of the world. We want children to feel confident, competent and happy mathematicians so they can enjoy the challenge and reward that maths offers.
How do we teach Maths at Freeland Primary School?
Teaching and Learning
We use our calculation policy, which is based on the White Rose Hub scheme of learning, to effectively teach children. This calculation policy makes use of the concrete, pictorial and abstract methodology which caters to all styles of learners and stages of learning. We teach using interactive resources as well as physical manipulatives to enable children to unlock new, difficult and abstract concepts and embed previous learning.
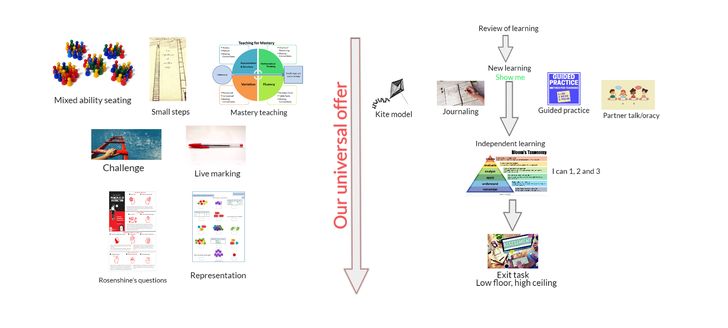
Learning is from previous lessons and topics reviewed at the beginning of lessons to ensure knowledge is embedded.
New learning is then carefully guided by the teacher to ensure all learners are supported and stretched
Independent tasks are planned using Bloom's taxonomy which supports as well as allows children to achieve greater depth, with more able children being offered rich and sophisticated problems, as well as exploratory, investigative tasks, within the lesson as appropriate. Practice and consolidation play a central role. Carefully designed variation within this builds fluency and understanding of underlying mathematical concepts. Teachers use precise questioning in class to test conceptual and procedural knowledge and assess children regularly to identify those requiring intervention, so that all children keep up. Teachers base their teaching on the 5 key principles of mastery.
Testing and misconceptions
We make use of regular, low level testing as well as summative assessment to enable teachers to forensically investigate children’s misconceptions and effectively teach gaps in their learning. This enables teaching to be dynamic and diagnostic and means that the curriculum is catered to individual year group and children’s needs.
Assessment data in maths is reviewed throughout the year to inform interventions and to also ensure that provision remains well-informed to enable optimum progress and achievement. End of year data is used to measure the extent to which attainment gaps for individuals and identified groups of learners are being closed. This data is used to inform whole school and subject development priorities for the next school year.
Planning and structure
We have created our own curriculum maps which ensures we are revisiting topics each term, giving children a chance to embed and build upon previous knowledge. We make use of the White Rose Hub resources as well as using resources from NCETM, PiXL and Deepening Understanding to aid mastery and deepen understanding.
Mathematical topics are taught in blocks, to enable the achievement of ‘mastery’ over time. This ensures that children are able to focus for longer on each specific area of Maths and develop a more secure understanding over time. This approach is also designed to enable children to progress to a greater depth of understanding.
Subsequent blocks continue to consolidate previous learning so that the children continually practise key skills and are able to recognise how different aspects of Maths are linked.
Times tables
Times tables are taught daily from year 2 onwards, by the end of year 4 children are expected to be fluent in timetables up to 12x in order to complete the statutory multiplication check. To aid children’s learning we use regular low stakes testing in class. We also make use of PiXL timestables app as homework to engage children to learn their times tables in different ways.
What is the impact of our teaching?
The school has a supportive ethos and our approaches support the children in developing their collaborative and independent skills, as well as empathy and the need to recognise the achievement of others. Regular and ongoing assessment informs teaching, as well as intervention, to support and enable the success of each child. These factors ensure that we are able to maintain high standards, with achievement at the end of KS2 above the national average and a high proportion of children demonstrating greater depth, at the end of each phase.
First Experiences/Building Blocks:
There are fundamental skills that it is important for children to develop the building blocks to future learning in maths, including that linked to calculation. These will be taught initially in EYFS/Year 1 but also revisited throughout KS1 and KS2 to ensure depth of understanding. These skills include:
- Ordinality – ‘the ordering of numbers in relation to one another’ – e.g. (1, 2, 3, 4, 5…)
- Cardinality – ‘understanding the value of different numbers’ – e.g. (7 = 17 = + 12 =
- Equality – ‘seven is the same value as four add three’ – e.g. =
- Subitising – ‘instantly recognizing the number of objects in a small group, without counting them’ – e.g. → five
- Conservation of number – ‘recognising that a value of objects are the same, even if they are laid out differently
- Counting on and back from any number – e.g. ‘five add three more totals eight’ ‘ten take away three totals seven’
- Using apparatus and objects to represent and communicate thinking
- Maths language – using mathematical words verbally in every-day situations – e.g. ‘climb up to the top’ / ‘climb down to the bottom’
The ability to calculate mentally forms the basis of all methods of calculation and has to be maintained and refined. A good knowledge of numbers or a ‘feel’ for numbers is the product of structured practise through progression in relevant practical maths experiences and visual representations.
By the end of Year 6:
Children will be equipped with efficient mental and written calculation methods, which they use with fluency. Decisions about when to progress should always be based on the security of pupils’ understanding and their readiness to progress to the next Step. At whatever Step in their learning, and whatever method is being used, children’s strategies must still be underpinned by a secure understanding and knowledge of number facts that can be recalled fluently.
The overarching aims are that when children leave Freeland CE Primary School they:
- Are able to recall number facts with fluency,
- Have developed conceptual understanding through being able to visualise key ideas – such as those related to place value - through experience with practical equipment and visual representations;
- Are confident to reason using the language of ‘support’ and ‘challenge’. Make use of diagrams and informal notes to help record steps and part answers when using mental methods that generate more information than can be kept in their heads;
- Have an efficient, reliable, written method of calculation for each number operation that they can apply with confidence when undertaking calculations that they cannot carry out mentally;
- Are able to make connections between all four number operations, understanding how they relate to one another, as well as how the rules and laws of arithmetic can be applied
How to Help Your Children at Home:
+ https://nrich.maths.org/primary